Blog

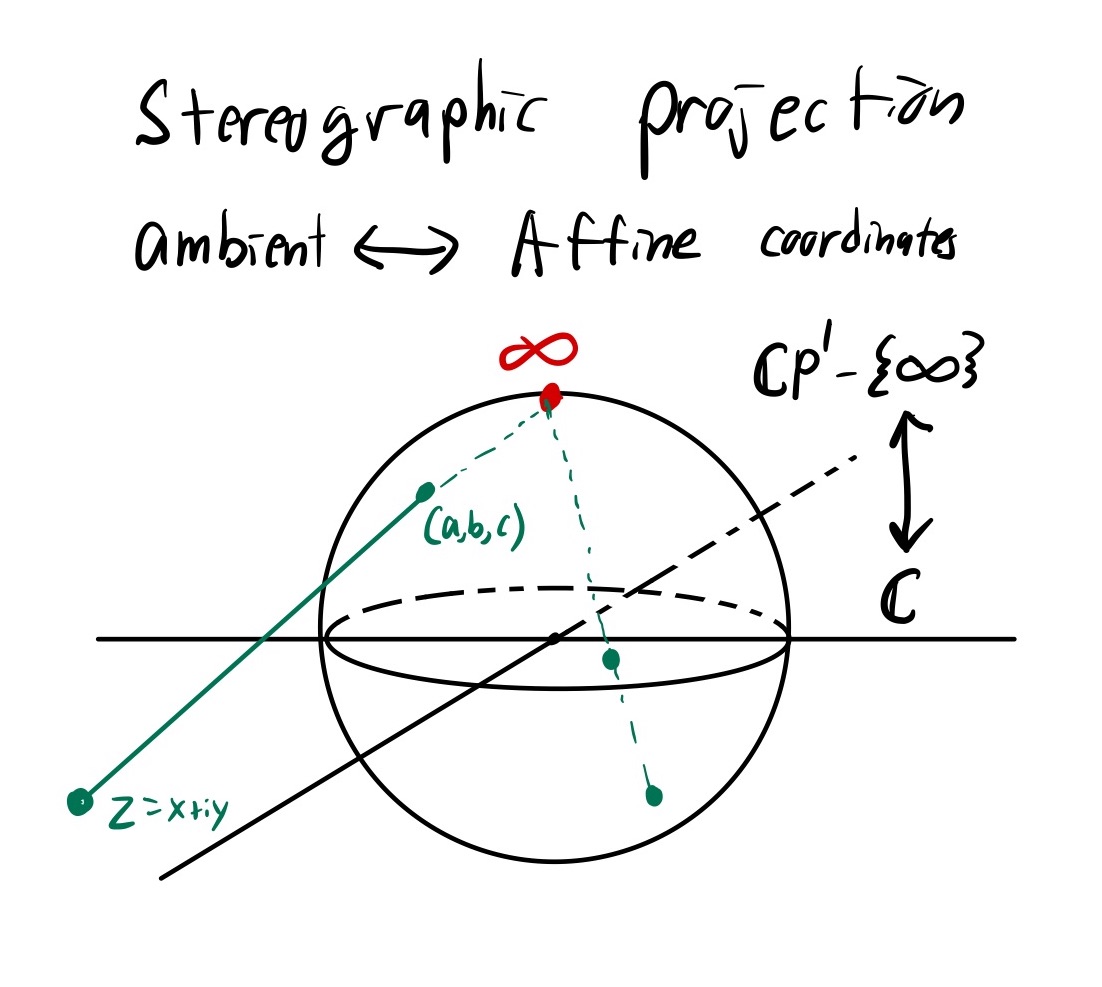
Fubini-Study boot camp

Summary:

A collection and coalittion of various facts and forms of the Fubini-Study form. I work out the kahler structure of $S^2$ in all of its different coordinates.

Spherical stick bombs

Summary:

Woven popsicle sticks store a large amount of elastic energy, held together by only friction. When thrown, the sticks can explosively fly apart, creating a stick bomb. Must stick bombs lie in a plane? Could you weave sticks along the surface of a sphere, making a rigid object without glue or bindings? What if you drop it?

Understanding hamiltonian G-spaces through quantization

Summary:

Philosophically, quantization converts functions on symplectic manifolds to opreators on hilbert spaces in a structure-preserving way. A symplectic manifold carrying a structure-preserving \(G\)-action quantizes to a \(G\)-action on the hilbert space, or a representation of \(G\). Using this, we can study representations through the geometry of symplectic manifolds. After outlining this philosophy, I describe a parralelism between symplectic manifolds and representations. Coadjoint orbits are irricudible representations, the moment map is a decomposition into irriducible representations. Constructions which engineer new representations become blueprints for building new symplectic manifolds. These constructions motivate hyperspherical varieties, the class of spaces starring in relative langlands duality

Presented at:

• UC Berkeley geometric representation theory seminar, fall 2023

Link to file

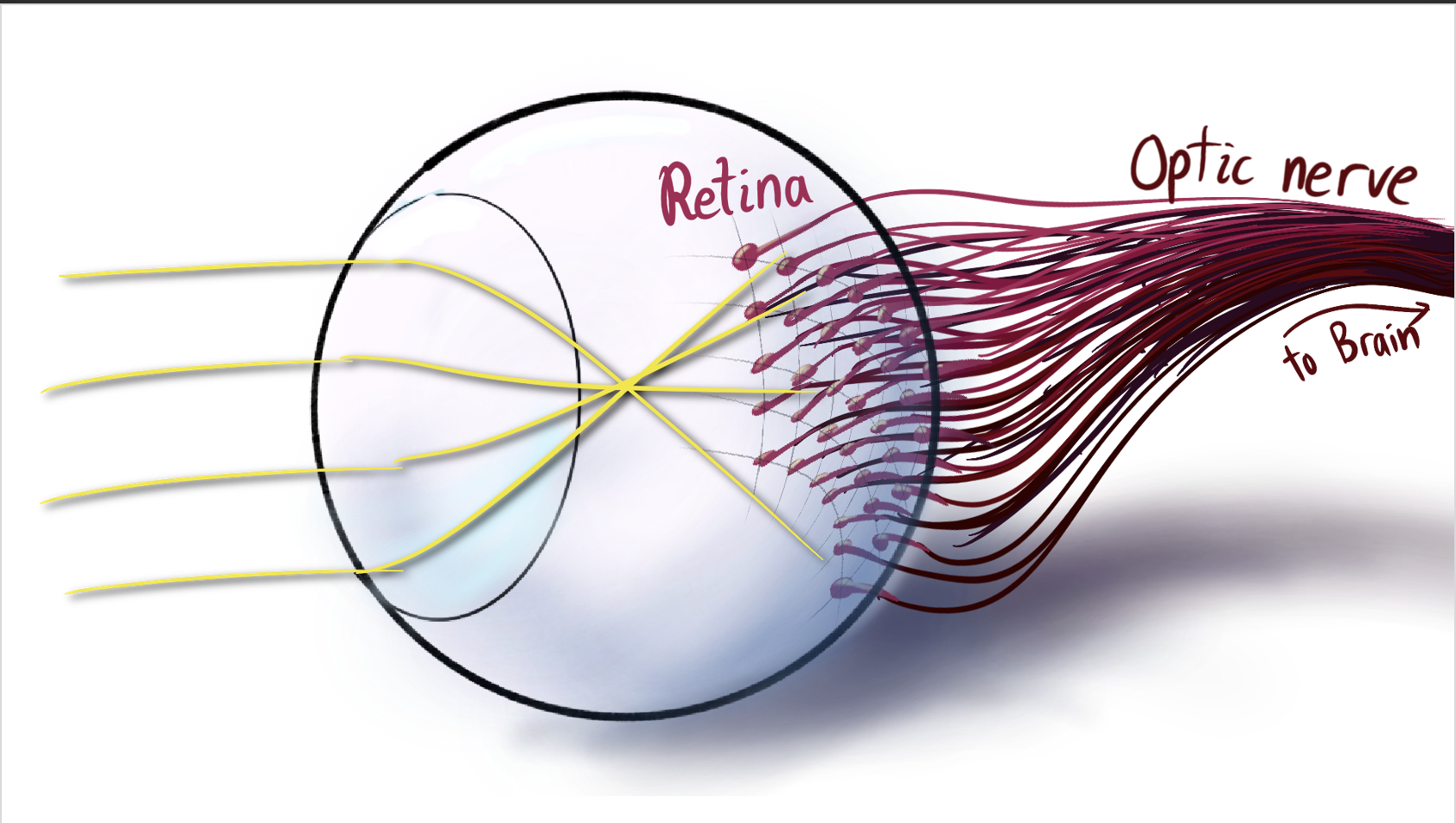
How we see with geometry

Summary:

When we see, the data from our eyes streams into the seemingly impenetrable jumble of neurons and synapses called our brain. In between, the signal passes through the visual cortex, a few layers of preprocessing which converts our visual field into abstract shapes. To do this, it seems evolution discovered differential geometry. First, the orientation of neurons in the visual cortex traces out a contact structure, a field of planes in R^3 tangent to no 2-dimensional submanifold. This automatically traces contours around everything we see. Second, the shape of the neurons themselves follow orbits of Euclidean and conformal symmetries, ensuring our perception is invariant under change of perspective. Together, this geometry will reveal itself to us through illusions and hallucinations.

Presented at:

• UC Berkeley Many cheerful facts, spring 2023

Link to file

Polygon moduli spaces

Summary:

The moduli space of polygons encodes how many ways you can bend the edges of a 3D polygon, ensuring the distance between adjacent points remains the same. In this talk, I gave people physical models of polygons, and had them figure out the moduli space up to rigid rotations. This reveals quite a lot of deep structure. Symplectic geometry, coadjoint orbits, toric geometry, and quiver varieties all come into play. We can even reframe the moduli space gauge theoretically as that of some flat connections on the punctured sphere. This web of equivalences make polygon spaces useful testing grounds. This is amplified when we pass to their hyperkahler analog, the hyperpolygon space, who connects with Nakajima quiver varieties and the moduli space of Higgs bundles.

Presented at:

• UC Berkeley geometric represenation theory seminar, Spring 2023

Hyperbolic string art

Summary:

I close my eyes, but all I see are strings. Stretch a line across a circle according to simple mathematical rules, and you get elegant patterns often dubbed “string art”. For example, connect each angle $\theta$ to the angle $2 \theta$, and the heart-shaped cardiod emerges. This talk chronicles my fourier into hyperbolic string art, a recontextualization of string art imagining the circle as the boundary of the hyperbolic plane, and the straight lines as hyperbolic geodesics. The patterns arising from natural hyperbolic transforms reveal the symmetries and geometry of hyperbolic space.  With hyperbolic string art, we navigate the hyperbolic plane watching only the horizon, and visualize the moduli space of closed hyperbolic surfaces.

Presented at:

• UC Berkerly many cheerful facts, Fall 2023

Link to file

Link to sketch

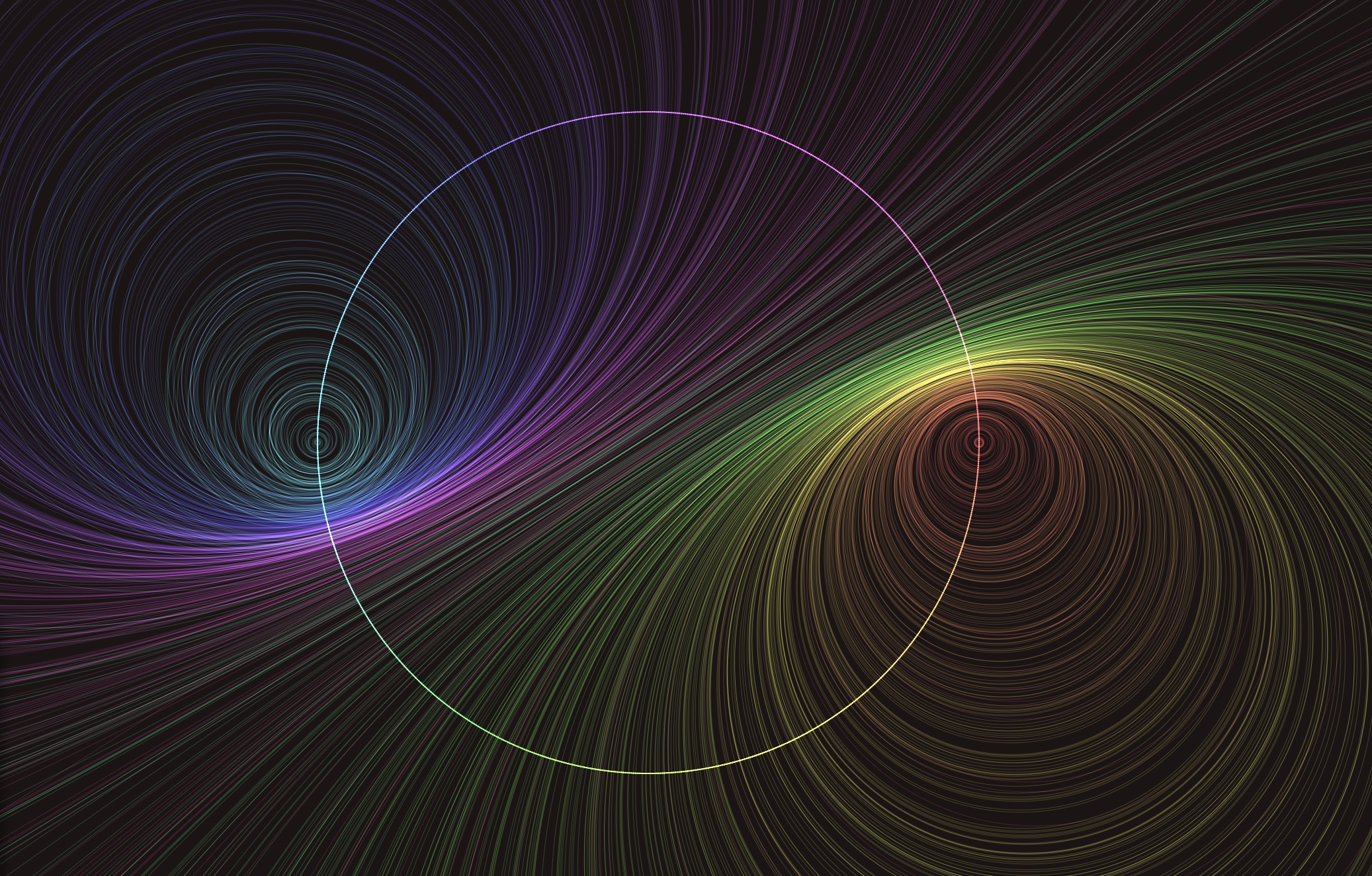
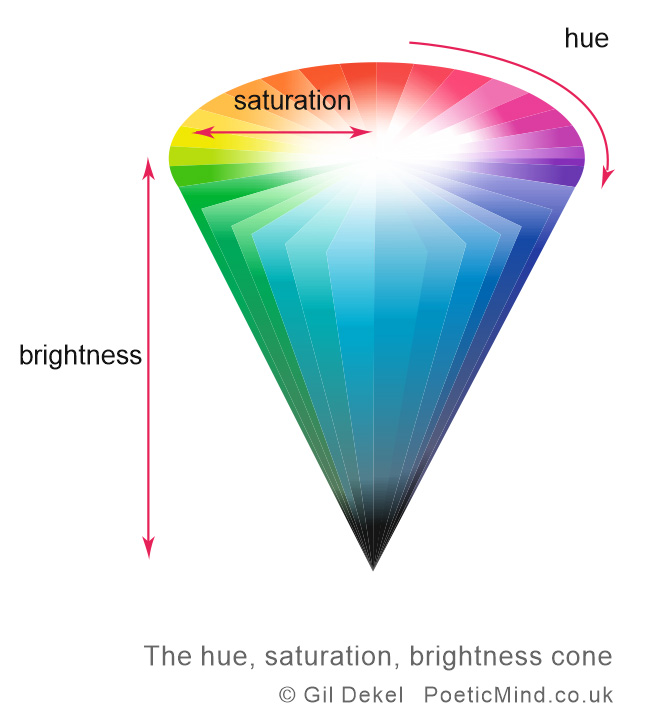
The shape of color

Summary:

Exploiting the psychologically of the brain, the space of colors carries a natural hyperbolic metric, turning the color wheel into a hyperbolic plane. Additive color mixing becomes hyperbolic translations, which work exactly like Lorentz boosts from general realtivity. That is, we can mix colors using spaceships :)

Toric geometry and harmonic oscillators

Summary:

In Spring 2022, my final project for symplectic geometry class was a blog post on toric geometry, which incorperated processing sketches with math exposition. This, of course, blew way out of proportion. This is what I’ve started. First, I give a quick explination of symplectic geometry motivated by classical mechanics. Second, a description of toric manifolds through the lens of harmonic oscilators. In particular, I explain the Dual axis illusion as a consequence of toric geometry.

Link to sketch

A Harbinger of Things to Come

Summary:

I haven’t been myself these past couple weeks. Maybe it’s the fall air, maybe quarantine has finally gotten to me, but I’ve betrayed one of my core values…